
Young mathematicians have been facing dismal job prospects throughout the nineties. The fall unemployment rate for new Ph.D.s in the U.S., as measured by the AMS-IMS-MAA Annual Survey (Second Report), rose from 2.5% in 1990 to a peak of 13.2% in 1994*. Unemployment rates have fallen moderately since to the current level of 9.5% in 1996. This is not the first time that labor market problems have plagued mathematics. The early seventies saw a similar situation. The Ph.D. glut of the seventies had far-reaching consequences. It led to drastic funding cutbacks in graduate education from which it took nearly 15 years to recover. The effects of the present labor market woes are already visible and dramatic, and they will certainly be damaging to mathematics in the long term. As we document below, the high unemployment rates facing recent Ph.D.s are only the tip of the iceberg.

A variety of external factors have contributed to the present situation. Changes in funding levels, recent immigration legislation, and the finances of higher education have all contributed to the present problems faced by Ph.D.s. It is all too easy to blame outside forces beyond our control for our troubles, however. The truth is that we in the mathematics community share the responsibility for the current employment crisis. Our community has dramatically expanded production of Ph.D.s without questioning whether there was sufficient demand for our product. Even after five years of serious and sustained employment problems, we have done little to adapt to the changes in the market for mathematicians.
Our community has failed to provide answers to the problems facing recent graduates. What is more, after five years we have barely begun asking the right questions in a systematic way. What are the effects of the current labor market problems on the mathematics community as a whole? What forces have contributed to these problems? What are effective remedies? We address each of these questions, providing partial answers when data exists, and pointing out the key gaps in our current understanding. We conclude by describing some specific steps that the mathematical societies can take to improve the current labor market situation for mathematics Ph.D.s.
The high unemployment rate of new Ph.D.s in the fall after graduation is a familiar fact in our community. However, the current labor market problems have had pernicious effects on all levels of mathematics, and these are considerably less well known. We first examine these effects to show just how damaging the labor market problems have been.
Consider the unemployment information presented in the AMS-MAA-IMS Annual Survey. The 8.1% reported unemployment rate is an important measurement, but it hides as much as it reveals. First of all, the Annual Survey figures systematically underestimate total unemployment rates by not taking into account unemployment among doctorates outside the U.S. The 8.1% figure is the ratio of unemployed doctorates known to be in the U.S. to the total number of doctorates whose whereabouts are known. If we compute instead the ratio of unemployed doctorates in the U.S. to the total number of doctorates known to be in the U.S., we obtain a more relevant U.S. unemployment rate of 9.5%.
The reported unemployment rate is distorted by a second factor as well. Some departments provide a form of welfare for Ph.D.s, offering temporary positions to graduates who are unable to find work elsewhere. It is not known how widespread this practice is, but the fact that nearly one quarter of Ph.D.s hired by U.S. doctoral degree-granting programs in 1996 (6.5% of all Ph.D.s known to be in the U.S.) were hired by the departments that granted them their degrees is telling. Furthermore, 3.8% of the employed Ph.D.s were working part-time, with at least 20% of these part-time employees still looking for full-time work. Even in the improved conditions of 1996, on the order of 16% of Ph.D.s in the U.S. were either unemployed or underemployed, twice the reported rate of unemployment.
The decrease in the U.S. unemployment rate from 12.8% in 1995 to 9.5% in 1996 is certainly encouraging. However, the simplest explanation for the fact that 38 fewer Ph.D.s were still looking for work in the U.S. in the fall of 1996 than in 1995 is that there were 73 fewer Ph.D.s granted in 1996 than 1995.
Little is known about what happens to Ph.D.s beyond the first year after obtaining their degrees. In 1995 the AMS conducted a study of the employment status of the class of 1991 two years after they obtained their degrees. In the fall after their graduation, 6.1% of the 1991 Ph.D.s in the U.S. were unemployed. Those who obtained short-term positions had a much harder time during their second round of job seeking. Of the 1991 Ph.D.s employed in U.S. academic institutions who changed jobs, 20% were unemployed in the fall of 1993. There has been no follow-up on this disturbing finding.
175 years ago economist David Ricardo observed, "labor is dear when it is scarce and cheap when it is plentiful." Not surprisingly, an 8% decline in real 9-month teaching and research salaries for new Ph.D.s has accompanied the increase in Ph.D. supply between 1989 and 1996. Moreover, a more subtle change is occurring. There is a hidden downward trend in total compensation for new Ph.D.s that is occurring as the types of jobs held by new Ph.D.s change. New Ph.D.s in academia are increasingly employed as temporary rather than tenure-track employees. Between 1990 and 1995, the number of full-time non-tenure-eligible faculty in traditional math departments (Groups I-III) increased by 37%. At the same time the number of tenure-track faculty fell by 27%. Temporary faculty now comprise 56% of all non-tenured faculty in traditional math departments.
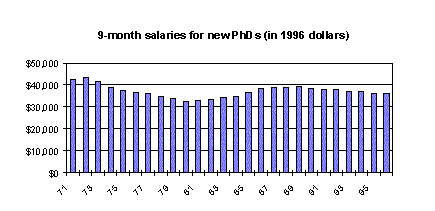
In addition to having no job security, temporary workers receive fewer benefits than tenure-track employees do. Furthermore, temporary employment delays entry onto the tenure-track salary ladder. Each year on postdoctoral-level wages delays the transition to assistant professor salary levels by one year, and results in one less year as a full professor. Thus, total lifetime earnings of new doctorates have been depressed.
An increase in the amount of time required to earn a Ph.D. represents a second form of reduction in lifetime earnings. National Research Council data show that the median time to degree* has increased from 6.5 to 8.0 years between 1982 and 1993. The current Ph.D. oversupply aggravates this problem by providing strong incentives for students to remain in graduate school for longer and longer periods of time in the hope that additional time for research will make them more marketable.
At present, no information is available on the average amount of time that new doctorates spend in temporary positions. Little is known about average total compensation for postdoctoral researchers or the effect of temporary positions on the time to tenure. Such information is essential for obtaining a true measure of the health of the profession.

The opportunity costs of graduate school have become increasingly difficult for prospective students to justify as the prospects and compensation for Ph.D.s decline and the time to degree increases. The median salary this year for new math Ph.D.s in 9-month teaching and research positions, the most common type of academic position held by new Ph.D.s, is $36,000. This is less than the $37,500 to $41,400 starting salaries commanded by 1996 bachelors degree recipients in electrical, computer, or chemical engineering. To our most talented students, the mere $6,000 difference in starting salary over that for mathematics bachelor’s degree holders does not make a strong economic case for years of intensive postbaccalaureate training amidst deteriorating employment conditions.
There is considerable evidence that labor market considerations play a strong role in determining educational and career choices for young people. In the words of Ed David, author of the David reports, "That [mathematics education is one of the best preparations for almost any career] may very well be true, but the students must believe that, or we won't have any students. And at the moment they don't appear to believe it." A recent AMS study bears this out. Applications to graduate programs in mathematics fell by 30% between the fall of 1994 and the fall of 1996. Moreover, the number of first year full-time graduate students in traditional math departments (Group I, II, and III schools) declined by roughly 23% between 1991 and 1996. The students we are losing are those with sufficient breadth of talent to pursue other opportunities. We are driving out intellectual diversity at precisely the time we need it most.
An anecdote of Harvard labor economist Richard Freeman puts these trends into perspective. Freeman was invited to speak to the physics department at the University of Chicago during the height of the physics employment crisis in the seventies. He writes,
What Freeman does not mention is his response to assertions such as those made by the chairman, something to the effect of, "Terrific. If that’s true, a 5% voluntary pay cut by senior scientists should be enough to prop up the market for entering physicists." This may have made his point.
University administrators are under considerable pressure to cut costs in the current climate of fiscal retrenchment in academia. In the past, cost-cutting mechanisms such as departmental downsizing, faculty wage freezes, and increased teaching loads have carried with them the risk of the loss of top faculty members and the inability to recruit new talent. Might long lines of talented, inexpensive, and job-hungry new doctorates standing ready to fill any available position embolden institutions to employ such measures? The recent attempts by the Regents of the University of Minnesota to eliminate tenure and by the University of Rochester to eliminate their mathematics graduate program are certainly suggestive.
The current job crunch for math Ph.D.s has two basic causes: a rapid increase in supply accompanied by a large decrease in demand. Both are important for understanding the present situation.
769 mathematics Ph.D.s were conferred in 1985. Ten years later, that number had grown to 1226, an increase of nearly 60%. The factors influencing departmental determinations of Ph.D. production levels have been examined in a series of faculty interviews conducted by William Massy of the Stanford Institute for Higher Education Research. Massy and co-author Charles Goldman report that
Massy and Goldman found that the primary factors used to determine Ph.D. program size are the number of faculty advisors available, the number of teaching assistants needed for staffing classes, the amount of research money available for funding assistantships, and the quality of the applicant pool. The recent increase in Ph.D. production has been driven by increases in two of these factors: funding levels and the size of the foreign applicant pool.
Federal support for the mathematical sciences increased by 34% in constant dollars between 1984 and 1989 following the release of the David Report in 1984. A substantial fraction of these new resources were used for funding graduate education. The David Report sought to reinstate funding for graduate education that was cut during the Ph.D. job crisis of the seventies. Ironically, in so doing, it has contributed to a repetition of the oversupply of Ph.D.s that led to the loss of funding in the first place.
A sizable increase in the foreign student population has also contributed to the expansion of Ph.D. production. The number of Ph.D.s granted to non-citizens nearly doubled between 1985 and 1995, and this increase has accounted for roughly two thirds of the growth in Ph.D. production over this time period. The presence of a large foreign student population in and of itself is no cause for concern. Indeed, a wide variety of international educational exchange programs have been designed to build ties between the scientific communities in U.S. and other countries, to promote cultural exchange, and to provide valuable training to the scientific workforce of less developed countries. Foreign exchange students who leave the U.S. after graduation have no impact on the U.S. labor market. The relevant question here is not how many non-citizen Ph.D.s are granted but how many of these students remain in the U.S. after graduation.
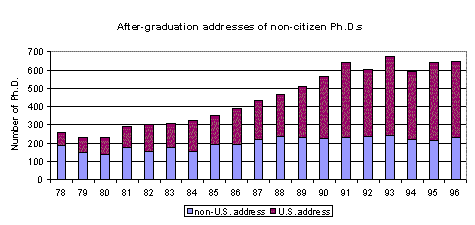
The Annual Surveys do not provide data on the post-graduation statuses of non-citizen doctorates. However, we can obtain a lower bound on the number in the U.S. in the fall after graduation by assuming that all new Ph.D.s known to be outside the U.S. are non-citizens. Figure 4 below compares the number of non-citizen Ph.D.s granted each year to the number of Ph.D.s known to be outside the U.S. in the fall after earning their degrees. At least 44% of non-citizen Ph.D.s were known to be in the U.S. in the fall after graduation in 1985. In contrast, the 1995 figure was 67%. Although the number of Ph.D.s granted to non-citizens has increased substantially over the past decade, the total number of new Ph.D.s employed outside the U.S. has remained nearly constant.
The influx of foreign Ph.D.s does not appear to be the sudden result of one-time political events such as the breakup of the Soviet Union and the post-Tiananmen Square exodus from China. On the contrary, as Figure 4 shows, the increase in the graduate non-citizen population has taken place gradually since the early eighties, well before these events. The Immigration Act of 1990 contains provisions, included at the behest of such organizations as the Association of American Universities to counteract projected Ph.D. shortages, which give university employers special privileges in hiring non-citizen faculty members. This legislation may well have contributed to an increase in immigration.
Data collected by the National Research Council show that perceived program quality, as measured by NRC faculty quality ratings, is strongly linked with program size. Out of seventeen objective departmental criteria measured, the study found the quantity most strongly correlated with perceived faculty quality was a measure of annual Ph.D.s production (r = 0.73). The correlation between faculty quality and the total number of students in the program is also relatively large (r = 0.63). The precise reason for this link is unknown. Perhaps a large program size, a "critical mass," is necessary to attract high quality faculty members. Having graduate students is viewed by faculty as a necessary condition for research productivity (and therefore program quality), and as a result faculty express strong resistance to the idea of decreasing enrollments within their own programs. Alternatively, perhaps having high faculty quality leads to expansion through increased access to grant money for funding students. In either case, the link between program size and perceived quality suggests that the drive for increased program quality may result in a system-wide tendency to expand Ph.D. production regardless of job market conditions.
While the supply of Ph.D.s continued to increase through the early nineties, demand fell. The number of positions offered in math departments declined by a third between 1989 and 1994. Much of this decrease can be attributed rapidly rising costs for higher education accompanied by cuts in government funding during that time period. Combined federal and state support for public higher education fell by 8.8% between 1980 and 1993 due in part to rising costs of Medicaid. Federal support for private institutions fell by 4% during the same period.
For the past several years, the science community has been tremulously following every nuance of the annual NSF budget negotiations. To be sure, these negotiations are important ones: NSF funding levels determine the availability of research assistants, summer salaries, and the speed of our computers. Even more important to our community is the financial health of the overall higher education system, yet to this central issue we pay relatively little attention.
Examination of the age distributions of mathematics departments shows a demographic bulge due to the large cohort of mathematicians hired during the late sixties and early seventies. The presence of this large cohort of mathematicians in their late fifties and early sixties, the recent elimination of mandatory retirement, and the current reduced hiring of junior tenure-track faculty all suggest that mathematics departments are aging. What are the effects of these shifting demographics? In a recent book, Professor Andrew Hacker puts it bluntly: "Every full professor who refuses to retire is preventing several young scholars from beginning their careers." We need to understand how departmental demographics are evolving and what the consequences of any changes will be.
Why have market forces not corrected the present job market problems? Market forces do appear to be in operation: first-year enrollments to graduate programs have fallen dramatically since the current job market woes began. The problem is one of timing. There is a lengthy delay between changes in first-year enrollments and the resulting changes in the Ph.D. supply. This type of delayed feedback system, called a "cobweb supply model", is commonly used in economics for studying markets for agricultural commodities. The result of the delay is oscillatory behavior in the system. When market conditions are good, enrollments increase. Many years later, these increased enrollments lead to an oversupply of Ph.D.s. The resulting poor market causes enrollments to fall, which leads to shortage conditions years later, and so on.
The period of the oscillation that results from the delayed feedback system is twice the amount of time between the decision to attend graduate school and the completion of a doctorate. Estimates of the median amount of time required to obtain a doctorate in mathematics range from 6.9 years to 8.0 years. There is an additional lag since the decision to attend graduate school must be made at least a year before enrollment to allow time for applying to schools. Hence this delayed feedback model predicts an oscillation in Ph.D. supply with a period of roughly 16 to 18 years. This is consistent with recent history: the Ph.D. supply peaked in the early seventies, bottomed out in the mid-eighties, and peaked again in the early nineties.
The discussion above suggests that an important factor in the current labor market problems is the way the supply of doctorates is currently regulated. The mathematical societies do not have the power to impose production quotas. Even if they did, such quotas would most likely create many more problems than they would solve. An important step that the societies can take instead is to provide sufficient information for prospective students, departments, and funding agencies to make more rational decisions regarding enrollments.
1. The mathematical societies should commission an annual report that analyzes trends affecting the supply of and demand for Ph.D.s five to ten years into the future.
If departments, students, and funding agencies are to make rational enrollment and funding decisions, they will need information about anticipated market conditions. The societies can help all three parties to make informed choices by providing an annual report outlining major trends affecting the supply and demand for Ph.D.s. This report should include a discussion of the effects of current and proposed legislation, demographic changes, political events abroad that affect immigration, trends in industry, and so on. The effort in preparing the report could be shared with scientific societies in other disciplines.
The report should be supplemented with projections of Ph.D. supply and demand. The time frame of the projections should be such that prospective students will have an idea about market conditions at the time of their graduation. Projecting supply over such a limited time frame is relatively straightforward given up-to-date information on current enrollment levels, attrition rates, and time to degree. See, for example, Freeman, 1989. Given the relatively strong historical correlation between the supply of Ph.D.s and the unemployment rate (r = 0.82), it is likely that supply estimates would prove to be quite valuable in assessing future market conditions.
Projecting demand is a much more difficult than projecting supply. The point, however, is not to provide a perfect forecast, but rather to provide informed estimates of the effects of various demand-side forces. For example, an estimate of the effects the recently passed five-year, $48 billion dollar tax incentive package for higher education on the demand for Ph.D.s in research-intensive versus teaching-intensive institutions would be quite valuable in assessing the need for training in teaching skills. The societies should draw upon outside expertise, especially that of labor market economists, in assessing the market conditions that will face new doctorates.
1(a) All analyses of supply and demand should be formulated in conjunction with a stringent review process to avoid potential conflicts of interest.
The notion that the supply of and demand for scientists and mathematicians can be predicted at all has been called into question by an infamous NSF study that projected a cumulative shortfall of 675,000 scientists and engineers between 1991 and 2006. A follow-up article by one of the study’s authors predicted a cumulative shortfall of 153,600 science and engineering Ph.D.s between 1995 and 2010. Despite strong criticism of the study’s methodology from experts both inside and outside the NSF, the study was broadly distributed to policy makers. Howard Wolpe, chairman of a 1992 congressional investigation into the release of the study, writes, "In 1987 the NSF adopted a plan to double its budget in five years. There is no doubt in my mind that this shoddy science was knowingly disseminated by the federal government’s premier scientific agency to further the attainment of this goal." Wolpe’s subcommittee found that criticism of the study "was ignored and even suppressed within the Foundation…. The NSF publications office … prevented the study from being printed as an official NSF document for over two years because of questions about credibility, until the director finally forced its publication." The lesson to be taken from the NSF study is not that the future is completely unforeseeable. Rather, it is that great care must be taken in light of the potential for serious conflicts of interest involved in projecting Ph.D. supply and demand.
1(b) The mathematical societies should re-evaluate the type of information collected in their annual departmental surveys. They should update assessments of future supply and demand regularly as new data becomes available.
In 1990 the David II Report recommended substantial expansions in mathematics Ph.D. production just months before the bottom fell out of the job market for new Ph.D.s. The report justified its recommendations using projections made by Bowen and Sosa.The trouble with these projections is that although they were carefully constructed and well documented, they relied on data and assumptions that were out of date. Several assumptions about Ph.D. production rates and immigration levels used by Bowen and Sosa were clearly wrong by the time of the David II Report’s release. For example, the 1987 Ph.D. production figures used for projecting future Ph.D. supply were old data, reflecting enrollment decisions made some 5 to 8 years earlier. First-year graduate enrollment figures reveal future changes in Ph.D. supply much more quickly than do graduation numbers. Had Bowen and Sosa had access to such enrollment data, their projections for the nineties may well have been quite different.
The lesson is that assessments of supply and demand need to be made on an ongoing basis, and these assessments should be revised as new information becomes available. Projections of future market conditions will require much more detailed data on attrition rates, time to tenure, departmental demographics, and the hiring of non-citizen doctorates than is currently collected. The societies should determine the data needs of supply and demand models and should adjust their data gathering accordingly. These data should be made public to facilitate research on the labor market for scientists.
The oversupply of Ph.D.s is not the only problem facing recent doctorates as they seek employment. A recent Bureau of Mathematical Sciences study of graduate programs found that "Many doctoral students are not prepared to meet undergraduate teaching needs, establish productive research careers, or apply what they have learned in business and industry." Furthermore, higher education is changing rapidly as student bodies become more heterogeneous in terms of ethnicity, income, age, and levels of student preparation. In a recent essay in the New York Times Magazine about these changes on campus, Professor Louis Menand writes,
What kinds of training programs are effective for various types of departmental missions? What is the best way to prepare students for careers at small liberal arts colleges? For careers at research universities? For careers in industry? If mathematics doctorates are to obtain employment of the type that they seek in the rapidly changing workplace, it is imperative that they receive the proper training. "My experience convinces me that graduate education can be changed to reflect the real needs of the profession, and the changes would not even have to be far-reaching. But we will have to be prepared to give up the idea that departments and schools have only minimum collective responsibility for the outcome," writes former Stanford president Donald Kennedy.
2. The mathematical societies should collect and make publicly available information on graduate placement rates for all Ph.D. programs.
A recent National Research Council report recommends that information on graduate placement rates for individual programs be gathered by the research community and made available on the Internet. Publicly available placement data would provide an invaluable measure of program effectiveness in preparing students for a wide variety of careers.
The outcome data collected for the new ratings would make it possible for the first time to evaluate program characteristics based on empirical considerations. For example, increasing the breadth of education has been widely advocated as a method for improving the job prospects of Ph.D.s. However, a number of mathematicians have raised concerns about the tradeoff between breadth and depth. Is breadth or depth more important, and in what contexts? This is a question best answered by looking at data on outcomes. Outcome data would serve to highlight a broad range of program characteristics that contribute to students’ preparation for successful careers.
Public outcome data would enable prospective students to make more informed choices about what graduate school to attend. They would steer students toward programs with graduate outcomes closely matching students’ own career aspirations. Outcome data would also provide students with realistic career expectations. Outcome data are but one factor among many for students to consider in choosing a graduate program. Other information that would be helpful includes data on time to degree, degree completion rates, and financial aid.
A key issue in the gathering placement data is that of how to assess graduate outcomes. The fact that a program’s graduates are employed does not indicate whether they are employed in jobs appropriate for their level of training. Who decides what is a positive graduate outcome? The answer is simple: we should turn to the graduates themselves for answers. Measures of the success of graduate outcomes should be based on responses of recent graduates to questions regarding their job satisfaction, the degree to which their training prepared them for their current positions, and the extent to which they use skills acquired in graduate training in their current positions. No value judgment needs to be made on the relative merits of industrial versus academic employment except by the doctorates themselves.
The environment in which mathematicians operate is changing rapidly. If our community is to govern itself in a responsible manner, it is imperative that we understand and adapt to these changes. Our reluctance to examine difficult issues such as the determination of enrollment levels, immigration, changing faculty demographics, and the effectiveness of various types of training programs neither makes these issues disappear nor mitigates their effects. The mathematical societies have the opportunity to take a strong leadership role here. Better information on the market for Ph.D.s and an assessment of the effectiveness of different types of training programs are not a cure-all prescription, but they do represent an important first step. We in the mathematics community need to take a more active role in solving our current labor market problems and in preventing future ones. The future of the profession and the next generation of mathematicians depend upon it.
The author would like to thank Sue Minkoff, Eric Weinstein, and many others for helpful feedback and comments. He also thanks the Digital Signal Processing group at Rice University for their generous hospitality during the writing of this paper.
(Note: Endnotes are available in the Acrobat, Postscript, and MS Word versions of this document)